1) Sketch the parabola, and lable the focus, vertex and directrix. a) (y - 1)^2 = -12(x + 4) b) i) y^2 - 6y -2x + 1 = 0, ii) y =
Por um escritor misterioso
Last updated 31 outubro 2024


9.2 Parabolas Emerald Seing. - ppt download

Conic Sections Parabolas Summary & Analysis

Find the vertex, focus, and directrix of each parabola. Grap
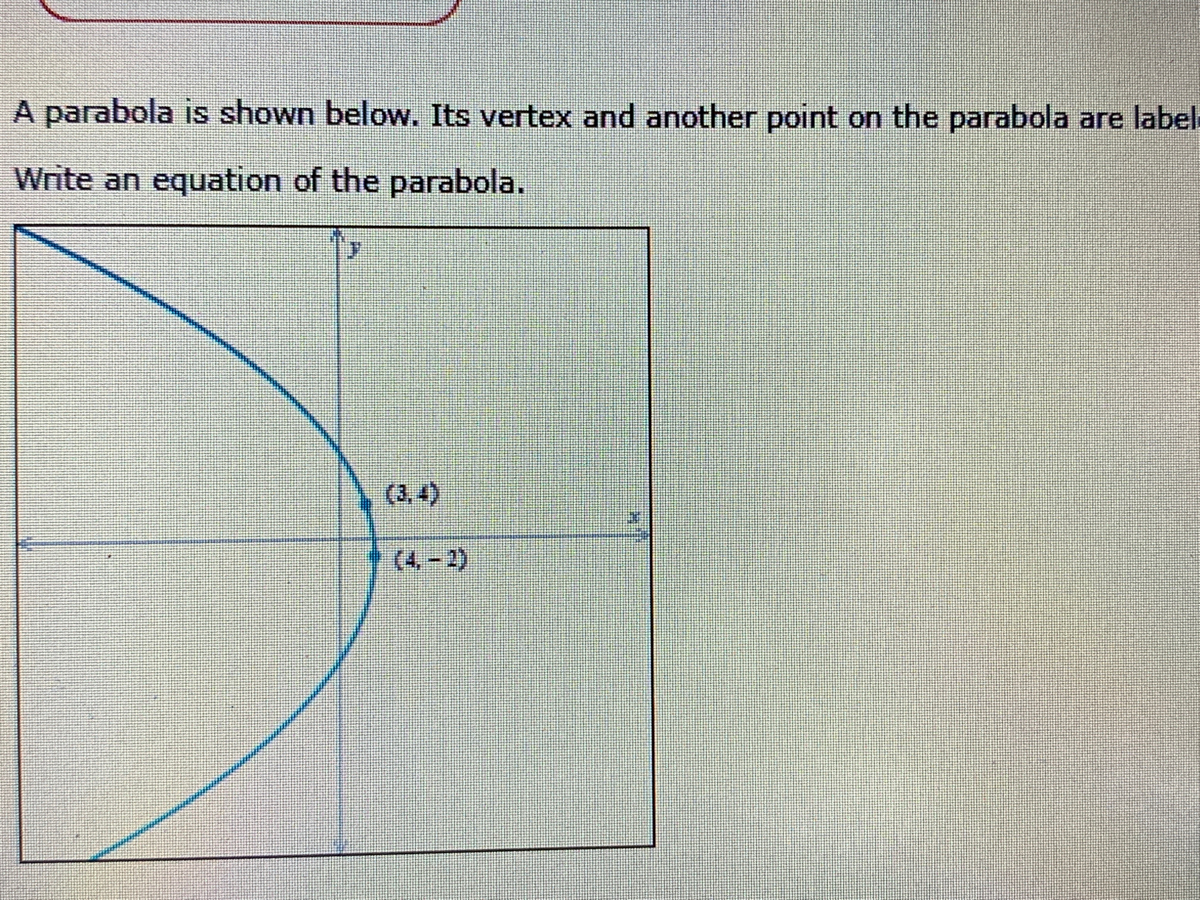
Answered: A parabola is shown below. Its vertex…

HELP! ONLY IF YOU KNOW THE ANSWER also this goes with the other question I asked Write the standard

SOLUTION: Maths exams - Studypool
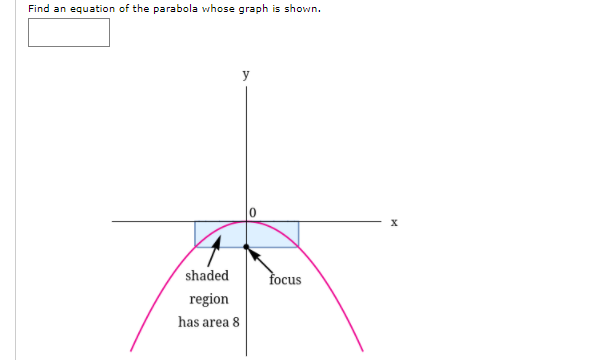
Answered: Find an equation of the parabola whose…
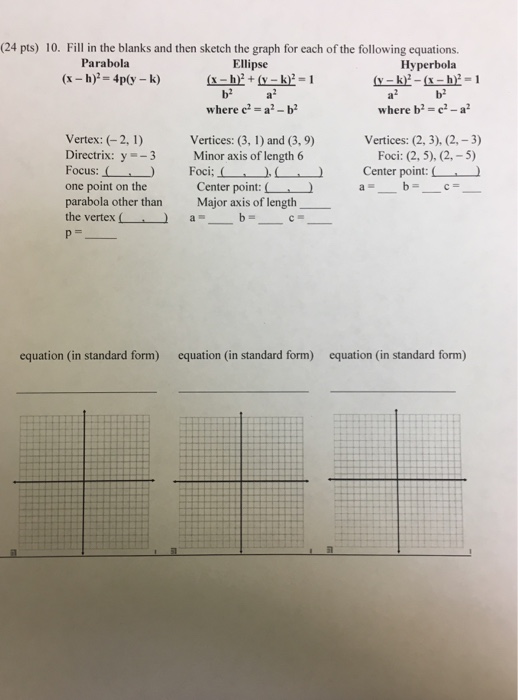
Solved Fill in the blanks and then sketch the graph for each
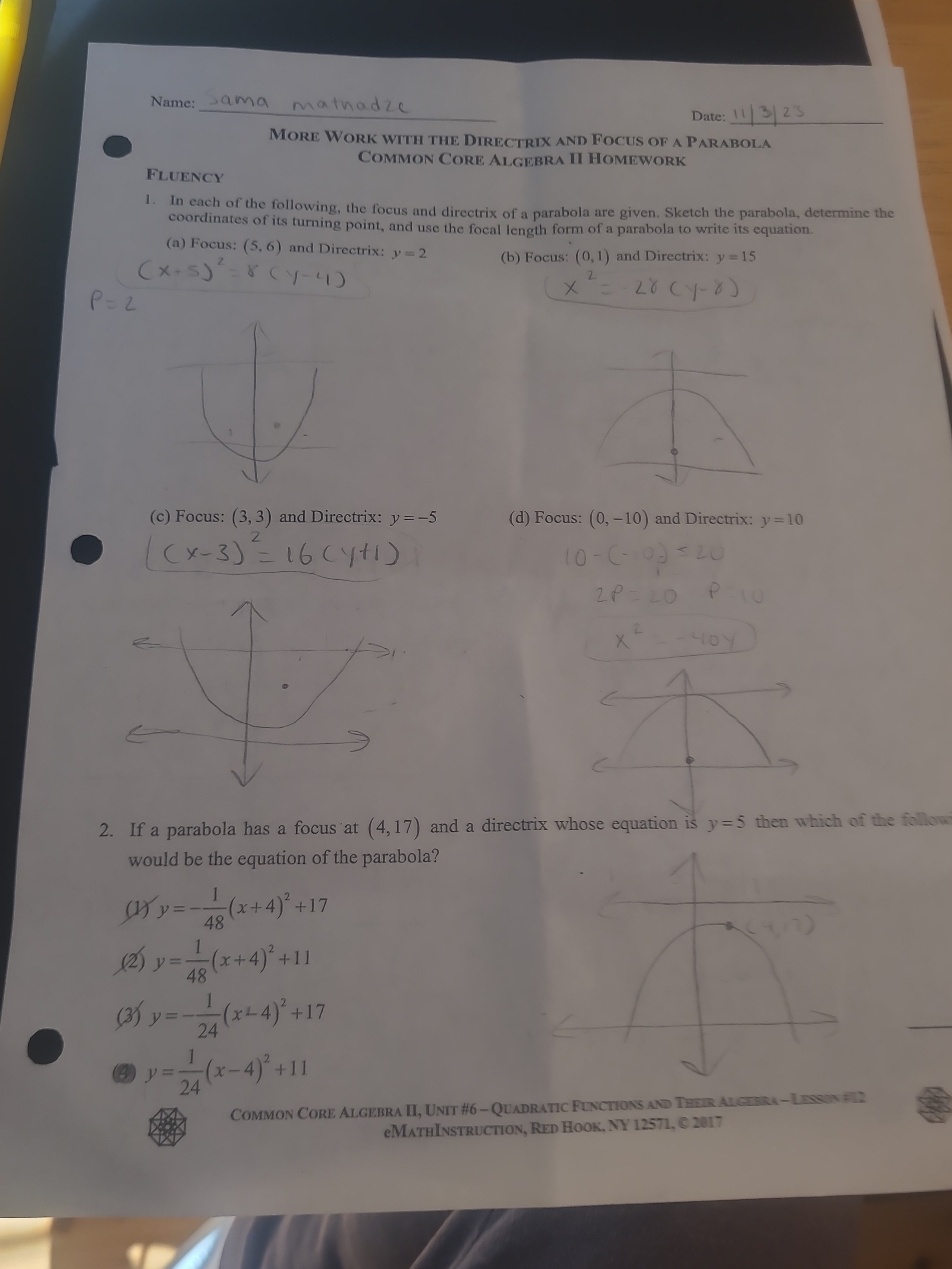
SOLVED: Name: sama mathadze Date: 11, 3

1 Lactus Rectum, PDF, Mathematical Objects

Conic sections: Analyzing Conic Sections with the Algebraic Method - FasterCapital
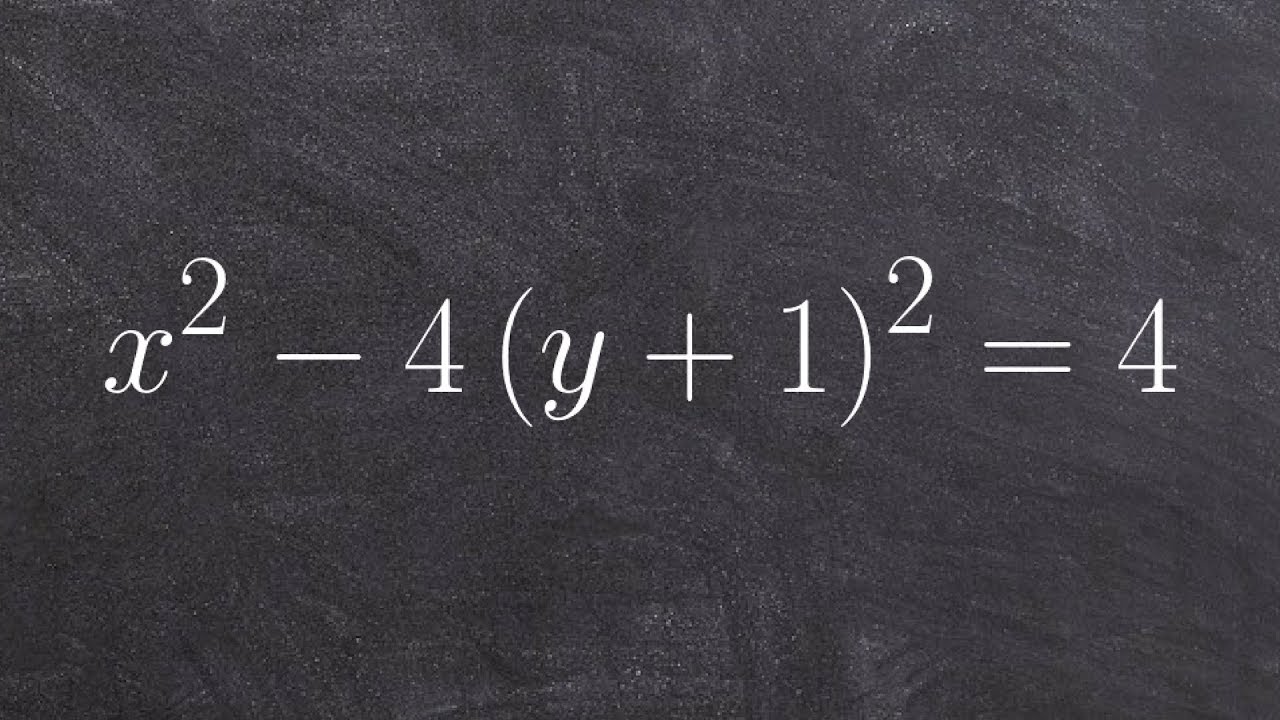
how to sketch the graph of a hyperbola in conic sections

Conic sections: Analyzing Conic Sections with the Algebraic Method - FasterCapital
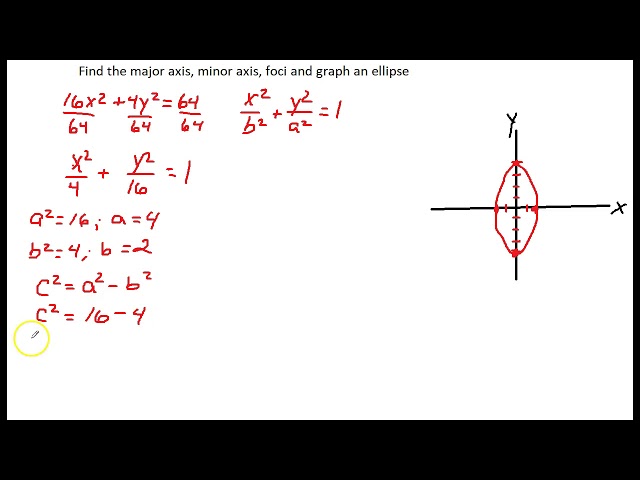
Find the major axis, minor axis, foci and graph an ellipse
Recomendado para você
-
 DirectX 12 (Ultimate) Download for Windows 10/11 PC - MiniTool31 outubro 2024
DirectX 12 (Ultimate) Download for Windows 10/11 PC - MiniTool31 outubro 2024 -
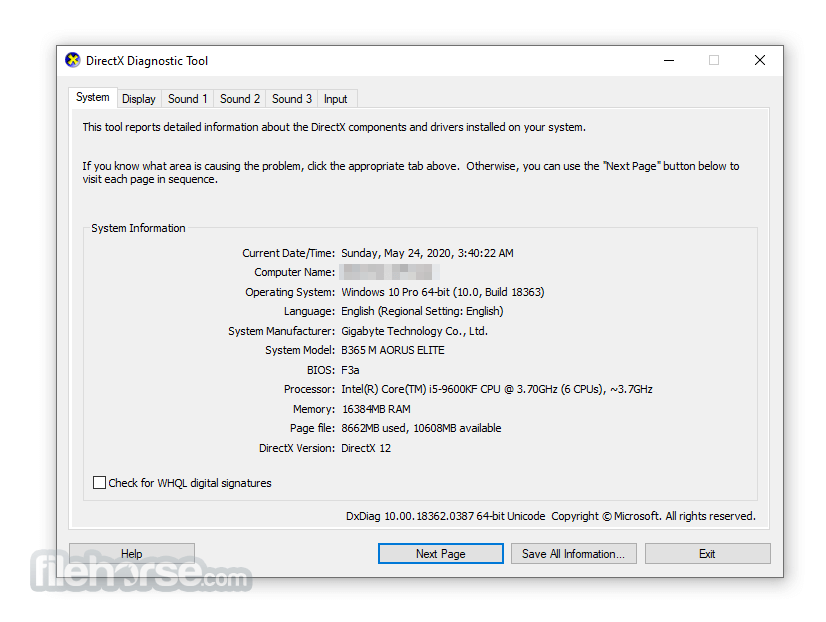 DirectX 12 Download (2023 Latest) for Win 11/10/731 outubro 2024
DirectX 12 Download (2023 Latest) for Win 11/10/731 outubro 2024 -
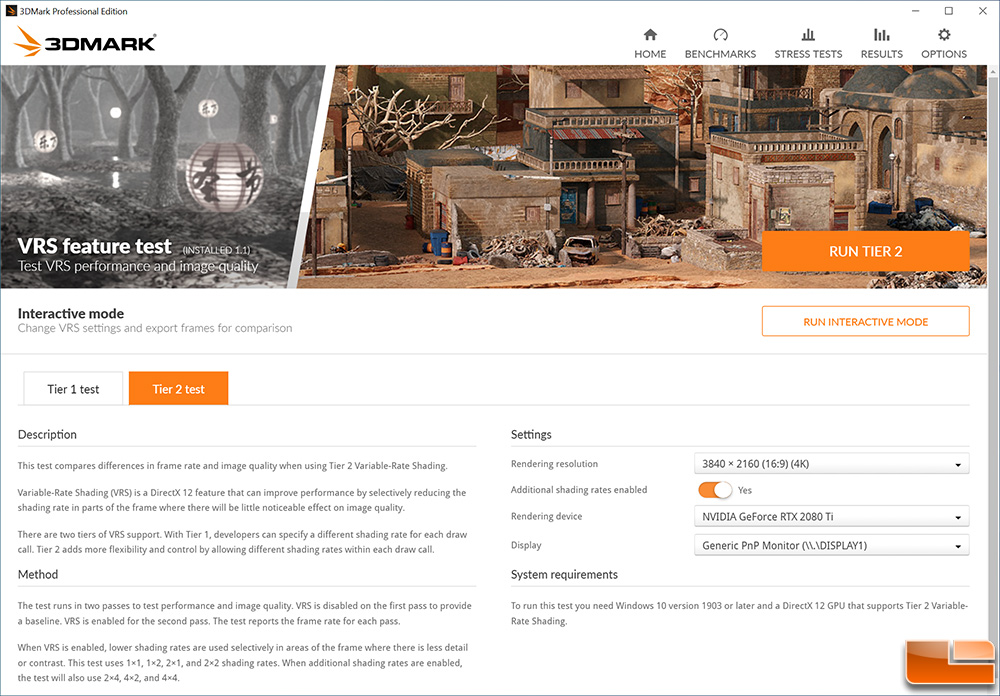 NVIDIA GeForce RTX 3090 Founders Edition Review - Page 9 of 15 - Legit Reviews31 outubro 2024
NVIDIA GeForce RTX 3090 Founders Edition Review - Page 9 of 15 - Legit Reviews31 outubro 2024 -
 Download DirectX Free - Latest Version 2023 ✓31 outubro 2024
Download DirectX Free - Latest Version 2023 ✓31 outubro 2024 -
directx 12 diagnostic tool display (directx12 Ultimate : disabled31 outubro 2024
-
 MSI GeForce GTX 1070 DirectX 12 GTX 1070 GAMING 8G 8GB 256-Bit GDDR5 PCI Express 3.0 x16 HDCP Ready SLI Support ATX Video Card31 outubro 2024
MSI GeForce GTX 1070 DirectX 12 GTX 1070 GAMING 8G 8GB 256-Bit GDDR5 PCI Express 3.0 x16 HDCP Ready SLI Support ATX Video Card31 outubro 2024 -
 Adaptador Directx Direct Box Tec731 outubro 2024
Adaptador Directx Direct Box Tec731 outubro 2024 -
 All NEW Fortnite Chapter 2 Settings! - DirectX 12, Console Motion Blur, & More!31 outubro 2024
All NEW Fortnite Chapter 2 Settings! - DirectX 12, Console Motion Blur, & More!31 outubro 2024 -
![Square Enix Unveils Impressive WITCH CHAPTER 0 [cry] DirectX 12 Tech Demo At Microsoft BUILD](https://imageio.forbes.com/blogs-images/marcochiappetta/files/2015/04/witch-cry-2.jpg?height=400&width=711&fit=bounds) Square Enix Unveils Impressive WITCH CHAPTER 0 [cry] DirectX 12 Tech Demo At Microsoft BUILD31 outubro 2024
Square Enix Unveils Impressive WITCH CHAPTER 0 [cry] DirectX 12 Tech Demo At Microsoft BUILD31 outubro 2024 -
Nancy Drew The Secret of Shadow Ranch - SHA - Manual, PDF, Menu (Computing)31 outubro 2024
você pode gostar
-
 Beth Roars31 outubro 2024
Beth Roars31 outubro 2024 -
:max_bytes(150000):strip_icc()/scarlet-fever-overview-1958805_fin-b03dd028ce63461c8bbdecef8eff4ff5.png) Scarlet Fever: What it Looks Like and What Causes it31 outubro 2024
Scarlet Fever: What it Looks Like and What Causes it31 outubro 2024 -
 E-voucher Presente - Pico Tango31 outubro 2024
E-voucher Presente - Pico Tango31 outubro 2024 -
 Xbox Game Studios Wallpaper 2020 by Playbox36 on DeviantArt31 outubro 2024
Xbox Game Studios Wallpaper 2020 by Playbox36 on DeviantArt31 outubro 2024 -
 Désiré - Prologue by Sylvain Seccia: Walkthrough & iOS iPad Air 2 Gameplay31 outubro 2024
Désiré - Prologue by Sylvain Seccia: Walkthrough & iOS iPad Air 2 Gameplay31 outubro 2024 -
 Shiny Genesect Pokemon eevee, Pokemon stories, Pokemon31 outubro 2024
Shiny Genesect Pokemon eevee, Pokemon stories, Pokemon31 outubro 2024 -
 Sala com plantas: conheça 7 espécies e saiba como decorar31 outubro 2024
Sala com plantas: conheça 7 espécies e saiba como decorar31 outubro 2024 -
 Akitaru Oubi31 outubro 2024
Akitaru Oubi31 outubro 2024 -
 Gacha Life, christmas, dolls, gacha club, gacha life, HD phone31 outubro 2024
Gacha Life, christmas, dolls, gacha club, gacha life, HD phone31 outubro 2024 -
 8 personagens desaparecidos que precisam retornar para Toy Story 5 - Nerd ao Quadrado31 outubro 2024
8 personagens desaparecidos que precisam retornar para Toy Story 5 - Nerd ao Quadrado31 outubro 2024